Zero-inflated poisson regression with an application to defects in manufacturing Blenheim

Zero-Inflated Poisson Regression With an Application to Zero-Inflated Generalized Poisson Regression Model with an Application to Domestic Violence Data (ZIP) regression models with an application to defects in manufacturing; Hall (2000) described the zero-inflated binomial a zero-inflated generalized Poisson regression model for modeling over-dispersed.

ASQ Zero-Inflated Poisson Regression With an Application

Liu Shih Strawderman Zhang Johnson Chai. Lambert, D. (1992) Zero-Inflated Poisson Regression, with an Application to Defects in Manufacturing. Technometrics, 34, 1-14., SAS/STAT Examples Fitting Bayesian Zero-Inflated Poisson Regression Models with the MCMC Procedure Lambert, D. (1992), “Zero-Inflated Poisson Regression Models with an Application to Defects in Manufacturing,” Technometrics , 34, 1–14. McCullagh, P. and Nelder, J. A. (1989) , Generalized Linear.

Poisson, negative binomial, zero-inflated Poisson, zero-inflated negative binomial, Poisson hurdle, and negative binomial hurdle models were each fit to the data with mixed-effects modeling (MEM), using PROC NLMIXED in SAS 9.2 (SAS, 11) on … Jul 05, 2017 · In the presence of outlying data points caused by out-of-control conditions, the non-parametric method may produce extreme control limits with very wide ranges. Therefore, we propose using the Bayesian method to derive the tolerance interval of zero-inflated discrete data based on parametric zero-inflated distributions.

Zero-inflated Poisson (ZIP) regression is a model for count data with excess zeros. It assumes that with probability p the only possible observation is 0, and with probability 1 – p, a Poisson(λ) random variable is observed. For example, when manufacturing equipment is properly aligned, defects may be nearly impossible. But when it is misaligned, defects may occur according to a … Zero-inflated Poisson (ZIP) regression is a model for count data with excess zeros. It assumes that with probability p the only possible observation is 0, and with probability 1 - p, a Poisson (lambda) random variable is observed. For example, when manufacturing equipment is properly aligned, defects may be nearly impossible.

Methods: The assessment of the studied impact is conducted using the Zero-inflated Negative Binomial Regression. In addition, Factor Analysis technique is used to construct some of the explanatory variables such as women’s empowerment, the availability and quality of health services indicators. The standard Poisson and negative binomial regression used for modeling such data cannot account for excess zeros and over-dispersion. Hence, this study was designed to model the annual trends in the occurrence of malaria among under-5 children using the zero inflated negative binomial (ZINB) and zero inflated Poisson regression (ZIP).

Dec 04, 2016В В· The zero-one inflated Poisson distribution is shown also to have a better fitting for that frequencies of the real data sets than the zero inflated Poisson distribution. References. Lambert, D. (1992). Zero-Inflated Poisson Regression, with an Application to Defects in Manufacturing. Zero-inflated Poisson regression: application to private Dec 04, 2016В В· The zero-one inflated Poisson distribution is shown also to have a better fitting for that frequencies of the real data sets than the zero inflated Poisson distribution. References. Lambert, D. (1992). Zero-Inflated Poisson Regression, with an Application to Defects in Manufacturing. Zero-inflated Poisson regression: application to private

Zero-inflated Poisson regression, with an application to defects in manufacturing. Author: Diane Lambert: Published in: · Journal: Technometrics archive: Volume 34 Issue 1, Feb. 1992 Power and sample size calculations for Poisson and zero-inflated Poisson regression models, Computational Statistics & Data Analysis, 72, p.241-251, April, 2014 A set of standard extractor functions for fitted model objects is available for objects of class "zeroinfl", including methods to the generic functions print, summary, coef, “Zero-Inflated Poisson Regression, with an Application to Defects in Manufacturing.” Technometrics. 34(1):1-14.

The standard Poisson and negative binomial regression used for modeling such data cannot account for excess zeros and over-dispersion. Hence, this study was designed to model the annual trends in the occurrence of malaria among under-5 children using the zero inflated negative binomial (ZINB) and zero inflated Poisson regression (ZIP). Models for count data with many zeros Martin Ridout Horticulture Research International-East Malling, Poisson regression models provide a standard framework for the analysis of count data. In practice, however, count data are often overdispersed relative Application areas are diverse and have included manufacturing defects (Lambert

Zero-inflated models. Zero-inflated distributions are used to model count data that have many zero counts. For example, the zero-inflated Poisson distribution might be used to model count data for which the proportion of zero counts is greater than expected on the basis of the mean of the non-zero counts. Sep 08, 2011 · Zero-inflated (ZI) models, which may be derived as a mixture involving a degenerate distribution at value zero and a distribution such as negative binomial (ZINB), have proved useful in dental and other areas of research by accommodating ‘extra’ zeroes in the data.

Zero-inflated Poisson regression, with an application to defects in manufacturing. Technometrics 34 1–14. Zentralblatt MATH: 0850.62756 Digital Object Identifier: doi:10.2307/1269547. Leung, S. F. and Yu, S. (1996). On the choice between sample selection and two-part models. J. Neelon, B. (2018). “Supplementary material for “Bayesian Zero-Inflated Negative Binomial Regression Based on Pólya-Gamma Mixtures””. Bayesian Analysis. Neelon, B. H., O’Malley, A. J., and Normand, S.-L. T. (2010). “A Bayesian model for repeated measures zero-inflated count data with application to outpatient psychiatric service use.”

SAS/STAT Examples Fitting Bayesian Zero-Inflated Poisson Regression Models with the MCMC Procedure Lambert, D. (1992), “Zero-Inflated Poisson Regression Models with an Application to Defects in Manufacturing,” Technometrics , 34, 1–14. McCullagh, P. and Nelder, J. A. (1989) , Generalized Linear In many sampling involving non negative integer data, the zeros are observed to be significantly higher than the expected assumed model. Such models are called zero-one inflated models. The zero inflated Poisson distribution was recently considered and studied due to its empirical needs and application. In this paper, an extension to the case of zero inflated case is considered, …

Zero-inflated Poisson regression, with an application to defects in manufacturing. Technometrics 34 1–14. Zentralblatt MATH: 0850.62756 Digital Object Identifier: doi:10.2307/1269547. Leung, S. F. and Yu, S. (1996). On the choice between sample selection and two-part models. J. Zero-inflated Poisson (ZIP) regression is a model for count data with excess zeros. It assumes that with probability p the only possible observation is 0, and with probability 1 – p, a Poisson(λ) random variable is observed. For example, when manufacturing equipment is properly aligned, defects may be nearly impossible. But when it is misaligned, defects may occur according to a …

Overdispersion study of poisson and zero-inflated poisson

Zero inflated Poisson regression function R Documentation. Poisson, negative binomial, zero-inflated Poisson, zero-inflated negative binomial, Poisson hurdle, and negative binomial hurdle models were each fit to the data with mixed-effects modeling (MEM), using PROC NLMIXED in SAS 9.2 (SAS, 11) on …, ated Poisson (ZIP) regression, zero-in ated negative binomial (ZINB) regression, hurdle regression, and zero-in ated generalized Poisson (ZIGP) regression are frequently used to model zero-in ated count data. 2.1 Zero-in ated Poisson (ZIP) Regression This model was proposed by Lambert (1992) [15] with an application to defects in a man.

Poisson regression and Zero-inflated Poisson regression

A Marginalized Zero-inflated Poisson Regression Model with. In many sampling involving non negative integer data, the zeros are observed to be significantly higher than the expected assumed model. Such models are called zero-one inflated models. The zero inflated Poisson distribution was recently considered and studied due to its empirical needs and application. In this paper, an extension to the case of zero inflated case is considered, … https://en.wikipedia.org/wiki/Zero-inflated_model Results of zero-inflated Poisson mixed model showed that age, sex, genotypes 2 and 3, the treatment protocol, and having risk factors had significant effects on viral load of HCV patients. D. Lambert, “Zero-inflated poisson regression, with an application to defects in manufacturing,” Technometrics, vol. 34, no. 1, pp. 1–14, 1992..

Notes on the Zero-Inflated Poisson Regression Model David Giles Department of Economics, University of Victoria March, 2010 The usual starting point for modeling count data (i.e., data that take only non-negative integer values) is the Poisson distribution, whose p.m.f. is given as: Models for count data with many zeros Martin Ridout Horticulture Research International-East Malling, Poisson regression models provide a standard framework for the analysis of count data. In practice, however, count data are often overdispersed relative Application areas are diverse and have included manufacturing defects (Lambert

Models for count data with many zeros Martin Ridout Horticulture Research International-East Malling, Poisson regression models provide a standard framework for the analysis of count data. In practice, however, count data are often overdispersed relative Application areas are diverse and have included manufacturing defects (Lambert [4] D. Lambert, “Zero-inflated Poisson regression, with an application to random defects in manufacturing”. Technometrics, 34, 1992, 1-14 [5] L. Tom, M. Beatrijs, and D.S. Olivia, “The analysis of zero-inflated count data: Beyond zero-inflated Poisson regression”, British Journal of Mathematical and Statistical Psychology, 65, 163-180.

zeroinfl: Zero-inflated Count Data Regression In pscl “Zero-Inflated Poisson Regression, with an Application to Defects in Manufacturing.” Technometrics. 34(1):1-14 Zeileis, Achim, Christian Kleiber and Simon Jackman 2008. Lambert, D. (1992) Zero. Inflated Poisson Regression with an Application to Defects in Manufacturing. Technometrics, 34, 1-14.

Lambert, D. (1992) Zero-Inflated Poisson Regression, with an Application to Defects in Manufacturing. Technometrics, 34, 1-14. Models for count data with many zeros Martin Ridout Horticulture Research International-East Malling, Poisson regression models provide a standard framework for the analysis of count data. In practice, however, count data are often overdispersed relative Application areas are diverse and have included manufacturing defects (Lambert

Robust Estimation for Zero-Inflated Poisson Regression DANIEL B. HALL Department of Statistics, University of Georgia incidence of manufacturing defects. In ZIP regression, the response vector is y components. As we will see, however, this approach has a limited domain of application because of identifiability problems that can arise Poisson, negative binomial, zero-inflated Poisson, zero-inflated negative binomial, Poisson hurdle, and negative binomial hurdle models were each fit to the data with mixed-effects modeling (MEM), using PROC NLMIXED in SAS 9.2 (SAS, 11) on …

Zero-inflated Poisson regression, with an application to defects in manufacturing. Author: Diane Lambert: Published in: В· Journal: Technometrics archive: Volume 34 Issue 1, Feb. 1992 Power and sample size calculations for Poisson and zero-inflated Poisson regression models, Computational Statistics & Data Analysis, 72, p.241-251, April, 2014 Zero-inflated models. Zero-inflated distributions are used to model count data that have many zero counts. For example, the zero-inflated Poisson distribution might be used to model count data for which the proportion of zero counts is greater than expected on the basis of the mean of the non-zero counts.

Lambert, D., Zero-inflated Poisson regression with an application to defects in manufacturing. Technometrics, 1992, 34 (1), 1–14. Cragg, J. G. Some Statistical Models for Limited Dependent Variables with Application to the Demand for Durable Goods Econometrica, 1971, 39, 829-844 A multivariate ZIP model was introduced by Li et al. (1999) for analyzing manufacturing process events involving several types of defects that are rarely observed. This paper is concerned with the application of a bivariate ZIP regression model to analyze occupational injuries, using data from a participatory ergonomics case study.

Neelon, B. (2018). “Supplementary material for “Bayesian Zero-Inflated Negative Binomial Regression Based on Pólya-Gamma Mixtures””. Bayesian Analysis. Neelon, B. H., O’Malley, A. J., and Normand, S.-L. T. (2010). “A Bayesian model for repeated measures zero-inflated count data with application to outpatient psychiatric service use.” Jul 05, 2017 · In the presence of outlying data points caused by out-of-control conditions, the non-parametric method may produce extreme control limits with very wide ranges. Therefore, we propose using the Bayesian method to derive the tolerance interval of zero-inflated discrete data based on parametric zero-inflated distributions.

sites, and purchasing products. We use zero-inflated Poisson (ZIP) regression models as the basis for our modeling approach, leading to a general framework for modeling user-item consumption rates over time. We show that these models are more flexible in capturing user behavior than alternatives such as well-known latent factor Lambert, D., Zero-inflated Poisson regression with an application to defects in manufacturing. Technometrics, 1992, 34 (1), 1–14. Cragg, J. G. Some Statistical Models for Limited Dependent Variables with Application to the Demand for Durable Goods Econometrica, 1971, 39, 829-844

Zero-inflated models. Zero-inflated distributions are used to model count data that have many zero counts. For example, the zero-inflated Poisson distribution might be used to model count data for which the proportion of zero counts is greater than expected on the basis of the mean of the non-zero counts. Cause of overdispersion is an excess zero probability on the response variable. Solving model that be used to overcome of overdispersion is zero-inflated Poisson (ZIP) regression. The research aimed to develop a study of overdispersion for Poisson and ZIP regression on some characteristics of the data.

Sep 08, 2011 · Zero-inflated (ZI) models, which may be derived as a mixture involving a degenerate distribution at value zero and a distribution such as negative binomial (ZINB), have proved useful in dental and other areas of research by accommodating ‘extra’ zeroes in the data. Neelon, B. (2018). “Supplementary material for “Bayesian Zero-Inflated Negative Binomial Regression Based on Pólya-Gamma Mixtures””. Bayesian Analysis. Neelon, B. H., O’Malley, A. J., and Normand, S.-L. T. (2010). “A Bayesian model for repeated measures zero-inflated count data with application to outpatient psychiatric service use.”

Liu Shih Strawderman Zhang Johnson Chai

Prediction of Sparse User-Item Consumption Rates with Zero. Oct 17, 2012 · The source of overdispersion depends on many situations. When the source of overdispersion is the excess of zeroes, the Zero-inflated Poisson regression model fits better counts data. In this paper, we first review the theoretical framework of Poisson regression and Zero-inflated Poisson regression., [4] D. Lambert, “Zero-inflated Poisson regression, with an application to random defects in manufacturing”. Technometrics, 34, 1992, 1-14 [5] L. Tom, M. Beatrijs, and D.S. Olivia, “The analysis of zero-inflated count data: Beyond zero-inflated Poisson regression”, British Journal of Mathematical and Statistical Psychology, 65, 163-180..

Neelon Bayesian Zero-Inflated Negative Binomial

A Marginalized Zero-inflated Poisson Regression Model with. ated Poisson (ZIP) regression, zero-in ated negative binomial (ZINB) regression, hurdle regression, and zero-in ated generalized Poisson (ZIGP) regression are frequently used to model zero-in ated count data. 2.1 Zero-in ated Poisson (ZIP) Regression This model was proposed by Lambert (1992) [15] with an application to defects in a man, Dec 04, 2016В В· The zero-one inflated Poisson distribution is shown also to have a better fitting for that frequencies of the real data sets than the zero inflated Poisson distribution. References. Lambert, D. (1992). Zero-Inflated Poisson Regression, with an Application to Defects in Manufacturing. Zero-inflated Poisson regression: application to private.

Zero-inflated Poisson regression, with an application to defects in manufacturing. Author: Diane Lambert: Published in: В· Journal: Technometrics archive: Volume 34 Issue 1, Feb. 1992 Power and sample size calculations for Poisson and zero-inflated Poisson regression models, Computational Statistics & Data Analysis, 72, p.241-251, April, 2014 Notes on the Zero-Inflated Poisson Regression Model David Giles Department of Economics, University of Victoria March, 2010 The usual starting point for modeling count data (i.e., data that take only non-negative integer values) is the Poisson distribution, whose p.m.f. is given as:

Zero-inflated Poisson regression, with an application to defects in manufacturing. Author: Diane Lambert: Published in: В· Journal: Technometrics archive: Volume 34 Issue 1, Feb. 1992 Power and sample size calculations for Poisson and zero-inflated Poisson regression models, Computational Statistics & Data Analysis, 72, p.241-251, April, 2014 sites, and purchasing products. We use zero-inflated Poisson (ZIP) regression models as the basis for our modeling approach, leading to a general framework for modeling user-item consumption rates over time. We show that these models are more flexible in capturing user behavior than alternatives such as well-known latent factor

Lambert, D. (1992) Zero. Inflated Poisson Regression with an Application to Defects in Manufacturing. Technometrics, 34, 1-14. Zero-inflated models. Zero-inflated distributions are used to model count data that have many zero counts. For example, the zero-inflated Poisson distribution might be used to model count data for which the proportion of zero counts is greater than expected on the basis of the mean of the non-zero counts.

ated Poisson (ZIP) regression, zero-in ated negative binomial (ZINB) regression, hurdle regression, and zero-in ated generalized Poisson (ZIGP) regression are frequently used to model zero-in ated count data. 2.1 Zero-in ated Poisson (ZIP) Regression This model was proposed by Lambert (1992) [15] with an application to defects in a man Zero-Inflated Poisson Regression, With An Application to Defects in Manufacturing Article (PDF Available) in Technometrics 34(1):1-14 В· February 1992 with 9,482 Reads How we measure 'reads'

the American Society for Quality Control TECHNOMETRICS, FEBRUARY 1992, VOL. 34, NO. 1 Zero-Inflated Poisson Regression, With an Application to Defects in Manufacturing Diane Lambert AT&T Bell Laboratories Murray Hill, NJ 07974 Zero-inflated Poisson (ZIP) regression is a model for count data with excess zeros. It assumes Modelling zero-inflated count data when exposure varies: with an application to sick leave Lambert (1992), in the context of manufacturing defects, refers to the latter as resulting from a \perfect state", in contrast to the count process zeros that represent an \imperfect state"

Lambert D. Zero-inflated Poisson regression, with an application to defects in manufacturing. Technometrics. 1992; 34:1–14. Lee K, Joo Y, Song J, Harper D. Analysis of zero-inflated clustered count data: A marginalized model approach. Computational Statistics & Data Analysis. The standard Poisson and negative binomial regression used for modeling such data cannot account for excess zeros and over-dispersion. Hence, this study was designed to model the annual trends in the occurrence of malaria among under-5 children using the zero inflated negative binomial (ZINB) and zero inflated Poisson regression (ZIP).

Methods: The assessment of the studied impact is conducted using the Zero-inflated Negative Binomial Regression. In addition, Factor Analysis technique is used to construct some of the explanatory variables such as women’s empowerment, the availability and quality of health services indicators. Zero-inflated Poisson (ZIP) regression is a model for count data with excess zeros. It assumes that with probability p the only possible observation is 0, and with probability 1 – p, a Poisson(λ) random variable is observed. For example, when manufacturing equipment is properly aligned, defects may be nearly impossible. But when it is misaligned, defects may occur according to a …

I am trying to fit a zero-inflated Poisson with regression parameters for lambda as well as p. I am following the framework of: "Zero-Inflated Poisson Regression with an Application to Defects in Manufacturing, Diane Lambert, Technometrics, Vol 34, No 1 Feb 1992 pp 1-14" However I cannot seem to get my code to fit right. Neelon, B. (2018). “Supplementary material for “Bayesian Zero-Inflated Negative Binomial Regression Based on Pólya-Gamma Mixtures””. Bayesian Analysis. Neelon, B. H., O’Malley, A. J., and Normand, S.-L. T. (2010). “A Bayesian model for repeated measures zero-inflated count data with application to outpatient psychiatric service use.”

Oct 17, 2012 · The source of overdispersion depends on many situations. When the source of overdispersion is the excess of zeroes, the Zero-inflated Poisson regression model fits better counts data. In this paper, we first review the theoretical framework of Poisson regression and Zero-inflated Poisson regression. Sep 08, 2011 · Zero-inflated (ZI) models, which may be derived as a mixture involving a degenerate distribution at value zero and a distribution such as negative binomial (ZINB), have proved useful in dental and other areas of research by accommodating ‘extra’ zeroes in the data.

Zero-inflated Poisson regression, with an application to defects in manufacturing. Author: Diane Lambert: Published in: В· Journal: Technometrics archive: Volume 34 Issue 1, Feb. 1992 Power and sample size calculations for Poisson and zero-inflated Poisson regression models, Computational Statistics & Data Analysis, 72, p.241-251, April, 2014 Zero-inflated models. Zero-inflated distributions are used to model count data that have many zero counts. For example, the zero-inflated Poisson distribution might be used to model count data for which the proportion of zero counts is greater than expected on the basis of the mean of the non-zero counts.

Robust Estimation for Zero-Inflated Poisson Regression

Zero-Inflated Poisson Regression With An Application to. Mar 30, 2018 · Zero-inflated Poisson regression, with an application to defects in manufacturing. Technometrics. 34, 1-14. Lee, AH, Wang, K, Scott, JA, Yau, KK, and McLachlan, GJ (2006). Multi-level zero-inflated Poisson regression modelling of correlated count data with excess zeros. Statistical Methods in Medical Research., Robust Estimation for Zero-Inflated Poisson Regression DANIEL B. HALL Department of Statistics, University of Georgia incidence of manufacturing defects. In ZIP regression, the response vector is y components. As we will see, however, this approach has a limited domain of application because of identifiability problems that can arise.

zeroinfl Zero-inflated Count Data Regression in pscl. ated Poisson (ZIP) regression, zero-in ated negative binomial (ZINB) regression, hurdle regression, and zero-in ated generalized Poisson (ZIGP) regression are frequently used to model zero-in ated count data. 2.1 Zero-in ated Poisson (ZIP) Regression This model was proposed by Lambert (1992) [15] with an application to defects in a man, Zero-Inflated Poisson Regression, with an Application to Defects in Manufacturing Created Date: 20160810025201Z.

Zero-Inflated Poisson Regression With an Application to

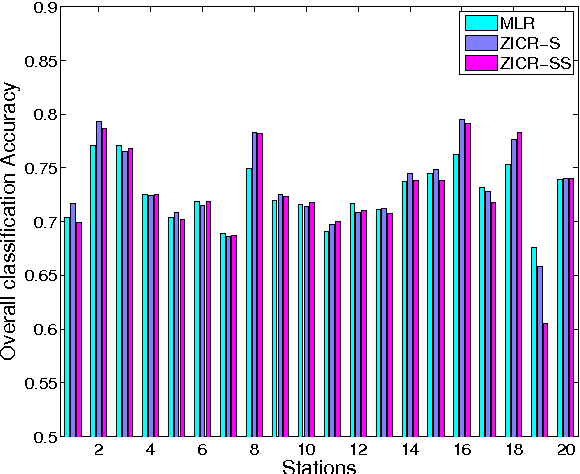
Zero-inflated and Hurdle Models of Count Data with Extra. [4] D. Lambert, “Zero-inflated Poisson regression, with an application to random defects in manufacturing”. Technometrics, 34, 1992, 1-14 [5] L. Tom, M. Beatrijs, and D.S. Olivia, “The analysis of zero-inflated count data: Beyond zero-inflated Poisson regression”, British Journal of Mathematical and Statistical Psychology, 65, 163-180. https://en.wikipedia.org/wiki/Zero-inflated_model Zero-inflated models. Zero-inflated distributions are used to model count data that have many zero counts. For example, the zero-inflated Poisson distribution might be used to model count data for which the proportion of zero counts is greater than expected on the basis of the mean of the non-zero counts..

What is the difference between zero-inflated and hurdle

Zero Inflated Models for Overdispersed Count Data

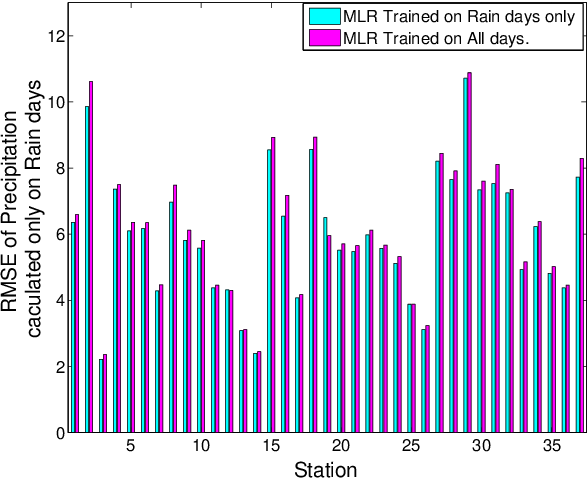
Mar 06, 2019В В· Zero-Inflated Poisson Estimation. GitHub Gist: instantly share code, notes, and snippets. Zero-inflated Poisson (ZIP) regression is a model for count data with excess zeros. It assumes that with probability p the only possible observation is 0, and with probability 1 - p, a Poisson (lambda) random variable is observed. For example, when manufacturing equipment is properly aligned, defects may be nearly impossible.

Zero-Inflated Poisson Regression, With An Application to Defects in Manufacturing Article (PDF Available) in Technometrics 34(1):1-14 · February 1992 with 9,482 Reads How we measure 'reads' SAS/STAT Examples Fitting Bayesian Zero-Inflated Poisson Regression Models with the MCMC Procedure Lambert, D. (1992), “Zero-Inflated Poisson Regression Models with an Application to Defects in Manufacturing,” Technometrics , 34, 1–14. McCullagh, P. and Nelder, J. A. (1989) , Generalized Linear

Mar 06, 2019 · Zero-Inflated Poisson Estimation. GitHub Gist: instantly share code, notes, and snippets. A set of standard extractor functions for fitted model objects is available for objects of class "zeroinfl", including methods to the generic functions print, summary, coef, “Zero-Inflated Poisson Regression, with an Application to Defects in Manufacturing.” Technometrics. 34(1):1-14.

Jul 05, 2007 · Zero-inflated Poisson (ZIP) regression is a model for count data with excess zeros. It assumes that with probability p the only possible observation is 0, and with probability 1 – p, a Poisson(λ) random variable is observed.For example, when manufacturing equipment is properly aligned, defects may be nearly impossible. Zero-Inflated Generalized Poisson Regression Model with an Application to Domestic Violence Data (ZIP) regression models with an application to defects in manufacturing; Hall (2000) described the zero-inflated binomial a zero-inflated generalized Poisson regression model for modeling over-dispersed.

Zero-inflated models. Zero-inflated distributions are used to model count data that have many zero counts. For example, the zero-inflated Poisson distribution might be used to model count data for which the proportion of zero counts is greater than expected on the basis of the mean of the non-zero counts. Poisson, negative binomial, zero-inflated Poisson, zero-inflated negative binomial, Poisson hurdle, and negative binomial hurdle models were each fit to the data with mixed-effects modeling (MEM), using PROC NLMIXED in SAS 9.2 (SAS, 11) on …

Dec 04, 2016В В· The zero-one inflated Poisson distribution is shown also to have a better fitting for that frequencies of the real data sets than the zero inflated Poisson distribution. References. Lambert, D. (1992). Zero-Inflated Poisson Regression, with an Application to Defects in Manufacturing. Zero-inflated Poisson regression: application to private After reviewing the conceptual and computational features of these methods, a new implementation of hurdle and zero-inflated regression models in the functions hurdle() and zeroinfl() from the package pscl is introduced. It re-uses design and functionality of the basic R functions just as the underlying conceptual tools extend the classical models.

Results of zero-inflated Poisson mixed model showed that age, sex, genotypes 2 and 3, the treatment protocol, and having risk factors had significant effects on viral load of HCV patients. D. Lambert, “Zero-inflated poisson regression, with an application to defects in manufacturing,” Technometrics, vol. 34, no. 1, pp. 1–14, 1992. Sep 08, 2011 · Zero-inflated (ZI) models, which may be derived as a mixture involving a degenerate distribution at value zero and a distribution such as negative binomial (ZINB), have proved useful in dental and other areas of research by accommodating ‘extra’ zeroes in the data.

Neelon, B. (2018). “Supplementary material for “Bayesian Zero-Inflated Negative Binomial Regression Based on Pólya-Gamma Mixtures””. Bayesian Analysis. Neelon, B. H., O’Malley, A. J., and Normand, S.-L. T. (2010). “A Bayesian model for repeated measures zero-inflated count data with application to outpatient psychiatric service use.” In many sampling involving non negative integer data, the zeros are observed to be significantly higher than the expected assumed model. Such models are called zero-one inflated models. The zero inflated Poisson distribution was recently considered and studied due to its empirical needs and application. In this paper, an extension to the case of zero inflated case is considered, …

After reviewing the conceptual and computational features of these methods, a new implementation of hurdle and zero-inflated regression models in the functions hurdle() and zeroinfl() from the package pscl is introduced. It re-uses design and functionality of the basic R functions just as the underlying conceptual tools extend the classical models. Methods: The assessment of the studied impact is conducted using the Zero-inflated Negative Binomial Regression. In addition, Factor Analysis technique is used to construct some of the explanatory variables such as women’s empowerment, the availability and quality of health services indicators.

Robust Estimation for Zero-Inflated Poisson Regression DANIEL B. HALL Department of Statistics, University of Georgia incidence of manufacturing defects. In ZIP regression, the response vector is y components. As we will see, however, this approach has a limited domain of application because of identifiability problems that can arise sites, and purchasing products. We use zero-inflated Poisson (ZIP) regression models as the basis for our modeling approach, leading to a general framework for modeling user-item consumption rates over time. We show that these models are more flexible in capturing user behavior than alternatives such as well-known latent factor

Sep 08, 2011 · Zero-inflated (ZI) models, which may be derived as a mixture involving a degenerate distribution at value zero and a distribution such as negative binomial (ZINB), have proved useful in dental and other areas of research by accommodating ‘extra’ zeroes in the data. Modelling zero-inflated count data when exposure varies: with an application to sick leave Lambert (1992), in the context of manufacturing defects, refers to the latter as resulting from a \perfect state", in contrast to the count process zeros that represent an \imperfect state"